Carnahan-Starling equation of state

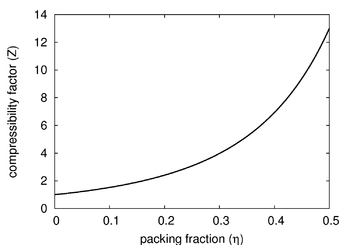
The Carnahan-Starling equation of state is an approximate (but quite good) equation of state for the fluid phase of the hard sphere model in three dimensions. It is given by (Ref Eqn. 10).

$Z={\frac {pV}{Nk_{B}T}}={\frac {1+\eta +\eta ^{2}-\eta ^{3}}{(1-\eta )^{3}}}.$

where:

• $Z$ is the compressibility factor
• $p$ is the pressure
• $V$ is the volume
• $N$ is the number of particles
• $k_{B}$ is the Boltzmann constant
• $T$ is the absolute temperature
• $\eta$ is the packing fraction:

$\eta ={\frac {\pi }{6}}{\frac {N\sigma ^{3}}{V}}$

• $\sigma$ is the hard sphere diameter.

The Carnahan-Starling equation of state is not applicable for packing fractions greater than 0.55 .

Virial expansion

It is interesting to compare the virial coefficients of the Carnahan-Starling equation of state (Eq. 7 of ) with the hard sphere virial coefficients in three dimensions (exact up to $B_{4}$, and those of Clisby and McCoy ):

$n$	Clisby and McCoy	$B_{n}=n^{2}+n-2$
2	4	4
3	10	10
4	18.3647684	18
5	28.224512	28
6	39.8151475	40
7	53.3444198	54
8	68.5375488	70
9	85.8128384	88
10	105.775104	108

Thermodynamic expressions

From the Carnahan-Starling equation for the fluid phase the following thermodynamic expressions can be derived (Ref Eqs. 2.6, 2.7 and 2.8)

Pressure (compressibility):

${\frac {p^{CS}V}{Nk_{B}T}}={\frac {1+\eta +\eta ^{2}-\eta ^{3}}{(1-\eta )^{3}}}$

Configurational chemical potential:

${\frac {{\overline {\mu }}^{CS}}{k_{B}T}}={\frac {8\eta -9\eta ^{2}+3\eta ^{3}}{(1-\eta )^{3}}}$

Isothermal compressibility:

$\chi _{T}-1={\frac {1}{k_{B}T}}\left.{\frac {\partial P^{CS}}{\partial \rho }}\right\vert _{T}-1={\frac {8\eta -2\eta ^{2}}{(1-\eta )^{4}}}$

where $\eta$ is the packing fraction.

Configurational Helmholtz energy function:

${\frac {A_{ex}^{CS}}{Nk_{B}T}}={\frac {4\eta -3\eta ^{2}}{(1-\eta )^{2}}}$

The 'Percus-Yevick' derivation

It is interesting to note (Ref Eq. 6) that one can arrive at the Carnahan-Starling equation of state by adding two thirds of the exact solution of the Percus Yevick integral equation for hard spheres via the compressibility route, to one third via the pressure route, i.e.

$Z={\frac {pV}{Nk_{B}T}}={\frac {2}{3}}\left[{\frac {(1+\eta +\eta ^{2})}{(1-\eta )^{3}}}\right]+{\frac {1}{3}}\left[{\frac {(1+2\eta +3\eta ^{2})}{(1-\eta )^{2}}}\right]={\frac {1+\eta +\eta ^{2}-\eta ^{3}}{(1-\eta )^{3}}}$

The reason for this seems to be a slight mystery (see discussion in Ref. ).

Kolafa correction

Jiri Kolafa produced a slight correction to the C-S EOS which results in improved accuracy :

$Z={\frac {1+\eta +\eta ^{2}-{\frac {2}{3}}(1+\eta )\eta ^{3}}{(1-\eta )^{3}}}.$

See also

• Equations of state for hard spheres
• Kolafa-Labík-Malijevský equation of state